In the realm of statistics, the Z critical value for 95 confidence interval holds immense importance, serving as a cornerstone in hypothesis testing, confidence interval construction, and decision-making processes. This comprehensive guide will delve deep into the intricacies of the Z value for 95 confidence, its calculation, practical applications, and the underlying principles that govern its usage.
Table of Contents
1. Introduction to Z Critical Value for 95 Confidence Interval
At its core, the 95 percent confidence interval critical value represents the number of standard deviations a data point deviates from the mean of a normal distribution. This fundamental concept underpins various statistical analyses, providing a standardized metric for assessing the significance of findings.
2. Understanding Confidence Intervals
Before delving into the specifics of the Z Critical Value for 95 Confidence Interval, it’s imperative to grasp the concept of confidence intervals. A confidence interval is a range of values within which an estimate is likely to fall. It provides a measure of certainty regarding the true value of a population parameter. Let’s explore different confidence intervals and their associated values.
Z Critical Value for 99% Confidence Interval
With a z critical value for 99% confidence interval, the z-value is approximately 2.576. This means that if the null hypothesis is true, the observed data point is 2.576 standard deviations away from the mean. The corresponding p-value is approximately 0.01, indicating a 1% chance of observing a result as extreme as the one obtained, assuming the null hypothesis is true.
Z Critical Value for 95% Confidence Interval
Z Critical Value for 95 Confidence Interval, the z-value is approximately 1.96. If the null hypothesis holds true, the observed data point is 1.96 standard deviations from the mean. The corresponding p-value is approximately 0.05, suggesting a 5% chance of observing a result as extreme as the one obtained, assuming the null hypothesis is true.
Z Critical Value for 90% Confidence Interval
For a z critical value for 90% confidence interval, the z-value is approximately 1.645. In this case, the observed data point is 1.645 standard deviations away from the mean, assuming the null hypothesis is true. The corresponding p-value is approximately 0.10, indicating a 10% chance of observing a result as extreme as the one obtained, assuming the null hypothesis is true.
Benefits of Confidence Intervals
Confidence intervals are crucial in statistical analysis as they provide a range of values that likely contain the true population parameter. This information helps researchers and decision-makers make informed choices, evaluate the reliability of their data, and conclude with a level of certainty.
3. Understanding P-Values
P-values are statistical measures used to determine the strength of evidence against a null hypothesis. They quantify the probability of obtaining results as extreme as those observed, assuming the null hypothesis is true.
Interpreting P-Values
The p-value associated with a confidence interval represents the likelihood of obtaining a result as extreme as the observed data point, assuming the null hypothesis is true. A decreased p-value suggests more compelling evidence for rejecting the null hypothesis.
4. Significance of Z Critical Value for 95% Confidence Interval
The choice of a 95 confidence interval critical value is rooted in statistical theory and practical considerations. A 95% confidence level implies that if we were to conduct the same experiment or sampling procedure multiple times, approximately 95% of the resulting confidence intervals would contain the true population parameter. This level of confidence strikes a balance between precision and reliability, making it a commonly used benchmark in statistical analysis.
5. Using Z Critical Value Tables
In practical applications, Z critical value tables serve as invaluable tools for determining critical values corresponding to different confidence levels and probabilities. These tables provide a quick reference for statisticians and researchers, facilitating the calculation of Z critical value with ease.
6. Z Critical Value for 95 Confidence Interval
The Z critical value of 1.96 for a 95% confidence interval is deeply ingrained in statistical practice. It signifies the boundary beyond which data points are considered statistically significant or lies within the confidence interval. Understanding this value is paramount for interpreting research findings, drawing conclusions, and making informed decisions based on sample data.
7. Importance in Statistical Analysis
The importance of the Z critical value cannot be overstated in statistical analysis. It forms the basis for hypothesis testing, where researchers assess the likelihood of observing a particular outcome under different scenarios. By comparing calculated test statistics with the Z critical value, researchers can determine the statistical significance of their results and make valid inferences about the underlying population.
8. Practical Examples
To illustrate the practical relevance of the Z critical value, consider a scenario where researchers are investigating the effectiveness of a new medical treatment. By calculating the Z critical value and comparing it with the observed treatment outcomes, researchers can ascertain whether the observed effects are statistically significant or merely due to chance.
9. Factors Influencing Z Critical Value
Several factors influence the Z critical value, including the desired confidence level, sample size, and the distribution of the data. Higher confidence levels require larger Z critical values, while larger sample sizes result in narrower confidence intervals and, consequently, smaller Z critical values.
10. Common Misconceptions
Despite its ubiquity in statistical practice, the concept of the Z critical value is often misunderstood. One common misconception is that the Z critical value remains constant across different sample sizes or confidence levels. In reality, the Z critical value varies depending on these factors and must be recalculated accordingly.
11. Tips for Efficient Calculation
Efficient calculation of the Z critical value is essential for streamlining statistical analyses and ensuring accuracy in results. Leveraging statistical software or online calculators can expedite the calculation process, particularly for complex analyses involving large datasets or multiple variables.
12. Advantages of Utilizing Z Critical Value
The utilization of the Z critical value confers several advantages in statistical analysis. By providing a standardized metric for assessing significance, the Z critical value facilitates comparability across studies, enhances reproducibility, and fosters a rigorous approach to data analysis.
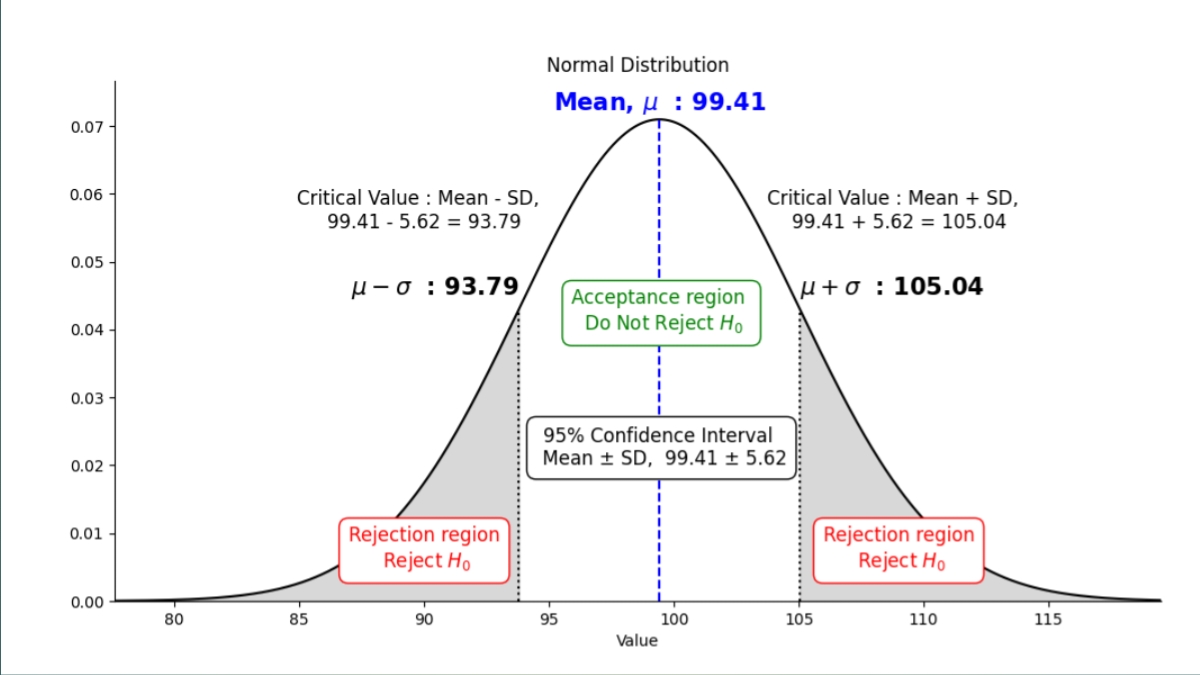
13. Example analysis
sample data = (87.6, 89.9, 91.6, 94.4, 95.7, 97.4, 97.6, 98.1, 98.2, 99.4, 99.8, 100.0, 101.7, 101.8, 102.2, 104.3, 105.4, 106.7, 107.0, 109.5)
The confidence interval reveals that we are 95% confident that the true population mean falls between 96.89 and 101.94. This indicates the range within which we expect the population mean to lie.
The p-value, which stands at 1.0, it’s a measure obtained from the statistical test. In essence, this value suggests that if the null hypothesis were true (assuming no significant difference), the observed data or even more extreme data would have been obtained with a probability of 1.0. In simpler terms, this high p-value implies that there isn’t enough evidence to reject the null hypothesis.
The standard deviation, hovering around 5.62, gives us insights into the variability or dispersion within the dataset. It essentially measures how much the individual data points deviate from the mean, offering a glimpse into the overall spread of the data.
14. Conclusion
In conclusion, the Z critical value for a 95% confidence interval serves as a linchpin in statistical analysis, providing researchers with a standardized metric for assessing significance and drawing valid conclusions from sample data. By understanding its calculation, interpretation, and practical implications, researchers can navigate the complexities of statistical inference with confidence and precision.
15. FAQs
Q1: Can the Z critical value be negative? A1: No, the Z critical value represents the number of standard deviations from the mean and, therefore, cannot be negative.
Q2: How does the confidence level affect the Z critical value? A2: Higher confidence levels necessitate larger Z critical values to encompass a greater proportion of the standard normal distribution.
Q3: Is the Z critical value the same as the Z-score? A3: While related, the Z critical value refers specifically to the threshold used in hypothesis testing and confidence interval construction, whereas the Z-score represents the number of standard deviations a data point is from the mean.
Q4: Why is a 95% confidence level commonly used? A4: A 95% confidence level strikes a balance between precision and reliability, offering a high degree of confidence while maintaining manageable margins of error.
Q5: Are there alternatives to the Z critical value for hypothesis testing? A5: Yes, alternatives include the t-distribution, particularly in cases where the population standard deviation is unknown or sample sizes are small.
Read more
2. How to Find P Value from t Statistic